Stochastic Partial Differential Equations:
I have been working on the maximal ![]() (or Sobolev)-regularity theory of SPDEs with the emphasis on the following:
(or Sobolev)-regularity theory of SPDEs with the emphasis on the following:
- Differential operators: 2nd-order operators, pseudo-differential operators, infinitesimal generator of Levy process, and operators related to anomalous diffusions.
- Domains:
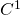 -domains, Lipschitz domains, angular domain and general non-smooth domains.
-domains, Lipschitz domains, angular domain and general non-smooth domains. - Coefficients: continuous leading coefficients, measurable leading coefficients, unbounded leading coefficients, and degenerate equations.
- Noises: Brownian motions, Levy noises, semi-martingales.
- Some quasi-and semi-linear SPDEs
2nd-oder Linear PDEs:
- Discontinuous, degenerate and unbounded leading coefficients.
- Weighted
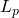 -regularity theory on non-smooth domains.
-regularity theory on non-smooth domains.
PDE related to stochastic processes:
I also have great interest in ![]() and H
and H![]() der regularity theory for PDEs whose differential operators are infinitesimal generator of stochastic processes such as Levy processes and non-stationary stochastic processes.
der regularity theory for PDEs whose differential operators are infinitesimal generator of stochastic processes such as Levy processes and non-stationary stochastic processes.
